Paul Lascabettes defends his thesis, Mathematical Models for the Discovery of Musical Patterns and Structures, and for Performances Analysis, at the Institut de Recherche et Coordination Acoustique / Musique (IRCAM, 1 place Igor-Stravinsky, 75004 Paris, France) at 2pm CET on Friday, 24 November 2023.
STMS Events announcement: https://www.stms-lab.fr/article-event-list
YouTube link for remote viewing of the defence: https://www.youtube.com/live/Agq2iZw1ZxY
Paul is a doctoral student at Sorbonne University under an ENS (Ecole normale supérieure Paris-Saclay) CDSN scholarship. He is also host of the highly successful Mathémusique YouTube channel. His PhD was carried out within the Musical Representations team of the STMS laboratory (Ircam – Sorbonne University – CNRS – French Ministry of Culture). He benefited from an EDITE doctoral contract. Paul’s thesis was supervised by Isabelle Bloch and Elaine Chew.



The defence will take place at Ircam, in English, before a jury comprising of :
- Isabelle BLOCH, Sorbonne University SIM (Sciences, Engineering, Medicine) – Thesis supervisor
- Elaine CHEW, King’s College London – Thesis co-director
- Mathieu GIRAUD, Centre de Recherche en Informatique, Signal et Automatique de Lille, CNRS – Rapporteur
- David MEREDITH, Department of Architecture, Design and Media Technology, Aalborg University – Rapporteur
- Moreno ANDREATTA, CNRS, IRMA – Examiner
- Gérard ASSAYAG, CNRS, STMS – Examiner
- Olivier LARTILLOT, RITMO, University of Oslo – Examiner
- Hugues TALBOT, CentraleSupélec – Examiner
Mathematical Models for the Discovery of Musical Patterns and Structures, and for Performances Analysis
Abstract: This manuscript focuses on the application of mathematical theories to music. In particular, we explore three closely related musical components: pattern, structure and performance, which can be associated with the following questions: How to discover musical patterns? How are these patterns organized within the musical structure? And finally, how are these patterns interpreted during musical performance?
First, this manuscript deals with the musical pattern discovery task. Previous works identify two different approaches: the sequential and the multidimensional approaches. We propose a method for developing the multidimensional approach. In particular, we demonstrate that the theory of mathematical morphology fits into this context. This makes it possible to obtain mathematical results for the discovery of musical patterns, and to provide answers to some of the open questions in the field.
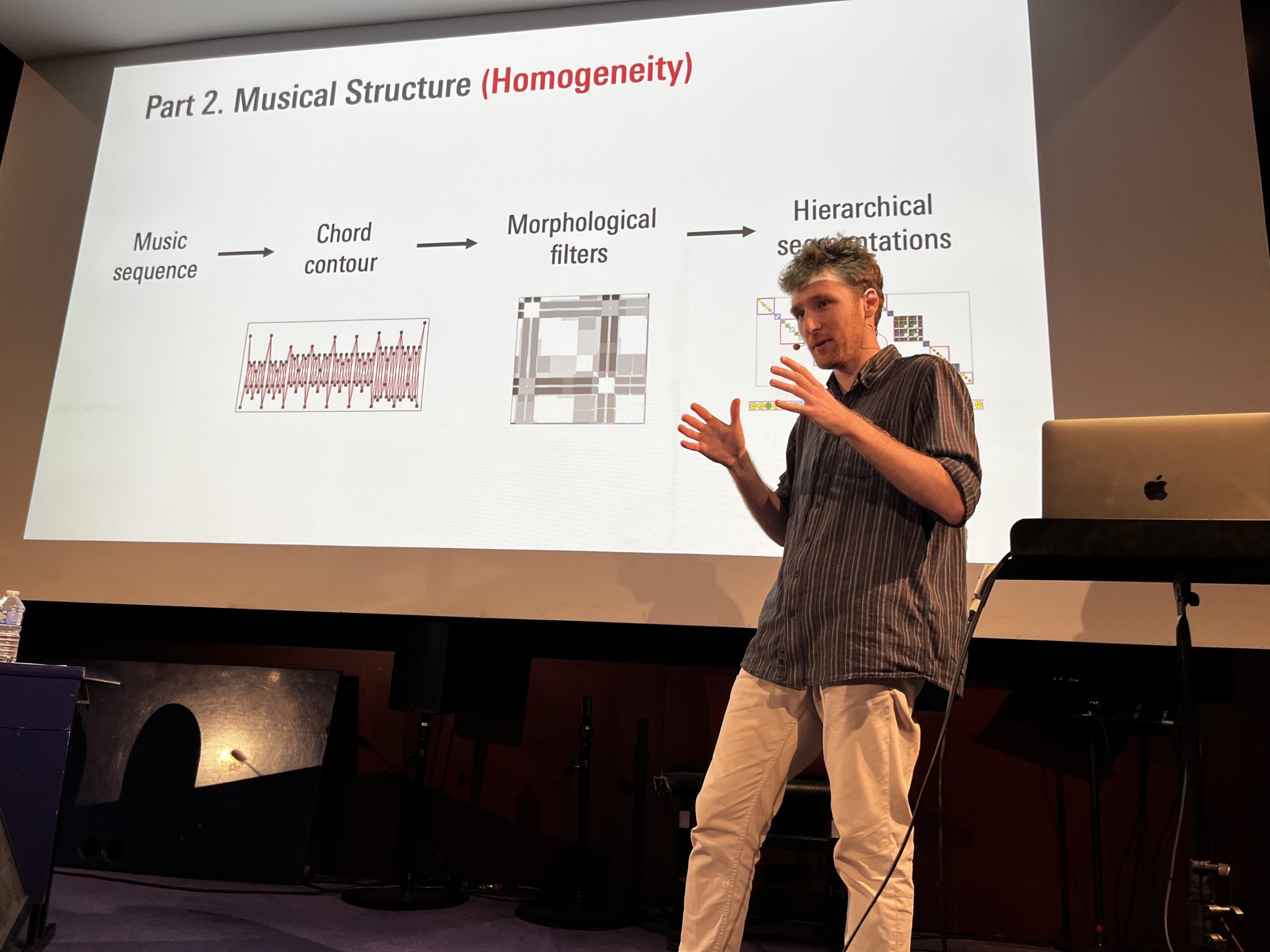
In the second part, we focus on the musical segmentation task, and we propose to generate hierarchical segmentations from a symbolic representation of music. We develop a method based on homogeneity and novelty, and one based on repetition, which are the main features to be studied in order to discover the segmentation of a piece. The homogeneity-based method uses morphological filters to detect blocks on the diagonal of the self-similarity matrix, while the repetition-based method discovers almost repetitions without overlaps to obtain the hierarchical segmentations of a musical piece.
The third part is dedicated to the computational models for music performances. We focus on the MazurkaBL dataset, which contains annotations of over 2000 recorded performances of 46 Chopin Mazurkas. To analyze this dataset, we propose to represent a musical performance in a 2-simplex, allowing us to characterize and interpret the expressivity of a performance. Then, we show that the theory of unbalanced optimal transport provides a tolerance to compare musical performances in order to identify similarities and differences between interpretations of the same piece.
Finally, we conclude this manuscript with a discussion of the application of such mathematical theories to music.
Keywords: Musical pattern discovery, mathematical morphology, music structure, music segmentation, musical performance